Related Topics: Common Core for Grade 7 Common Core for Mathematics Lesson Plans and Worksheets for all Grades More Lessons for Grade 7 Examples, solutions, worksheets, videos, and lessons to help Grade 7 students learn how to solve real-world and mathematical problems involving area, volume and surface area of two- and three-dimensional objects composed of triangles, quadrilaterals, polygons. Solve word problems involving volume and surface area involving 3D shapes, including composite figures. Math 7th grade Geometry Volume and surface area word problems. Volume and surface area word problems. Volume word problem: gold ring. Volume of triangular prism & cube.
Problem 1 :
Erin is making a jewelry box of wood in the shape of a rectangular prism. The jewelry box will have the dimensions shown below. The cost of painting the exterior of the box is $0.50 per square in. How much does Erin have to spend to paint the jewelry box ?
Problem 2 :
A metal box that is in the shape of rectangular prism has the following dimensions. The length is 9 inches, width is 2 inches, and height is 1 1/2 inches. Find the total cost of silver coating for the entire box.
Problem 3 :
Cherise is setting up her tent. Her tent is in the shape of a trapezoidal prism shown below. How many cubic feet of space are in her tent ?
Problem 4 :
Allie has two aquariums connected by a small square prism. Find the volume of the double aquarium.
Solutions
Problem 1 :
Erin is making a jewelry box of wood in the shape of a rectangular prism. The jewelry box will have the dimensions shown below. The cost of painting the exterior of the box is $0.50 per square in. How much does Erin have to spend to paint the jewelry box ?
Solution :
To know that total cost of painting, first we have to know the Surface area of the jewelry box.
Find surface area of the box.
Step 1 :
Identify a base, and find its area and perimeter.
Any pair of opposite faces can be the bases. For example, we can choose the bottom and top of the box as the bases.
Find base area.
B = l x w
B = 12 x 15
B = 180 square in.
Find perimeter of the base.
P = 2(12) + 2(15)
P = 24 + 30
P = 54 in.
Step 2 :
Identify the height, and find the surface area.
The height h of the prism is 6 inches. Use the formula to find the surface area.
S = Ph + 2B
S = 54(6) + 2(180)
S = 684 square inches
Step 3 :
Total cost = Area x Cost per square in.
Total cost = 684 x $0.50
Total cost = $342
So, Erin has to spend $342 to paint the jewelry box.
Problem 2 :
A metal box that is in the shape of rectangular prism has the following dimensions. The length is 9 inches, width is 2 inches, and height is 1 1/2 inches. Find the total cost of silver coating for the entire box.
Solution :
To know that total cost of silver coating, first we have to know the Surface area of the metal box.
Find surface area of the box.
Step 1 :
Identify a base, and find its area and perimeter.
Any pair of opposite faces can be the bases. For example, we can choose the bottom and top of the box as the bases.
Find base area.
B = l x w
B = 9 x 2
B = 18 square in.
Find perimeter of the base.
P = 2(9) + 2(2)
P = 18 + 4
P = 22 in.
Step 2 :
Identify the height, and find the surface area.
The height h of the prism is 1 1/2 inches. Use the formula to find the surface area.

S = Ph + 2B
S = 22(1 1/2) + 2(18)
S = 22(3/2) + 36
S = 33 + 36
S = 69 square inches
Step 3 :
Total cost = Area x Cost per square in.
Total cost = 69 x $1.50
Total cost = $103.50
So, the total cost of silver coating for the entire box is $103.50.
Problem 3 :
Cherise is setting up her tent. Her tent is in the shape of a trapezoidal prism shown below. How many cubic feet of space are in her tent ?
Solution :
Step 1 :
To find the number of cubic feet of space in the tent, we have to find the volume of Cherise's tent.
Step 2 :
Volume of Cherise's tent (Trapezoidal prism) is
= base area x height
or
V = b x h
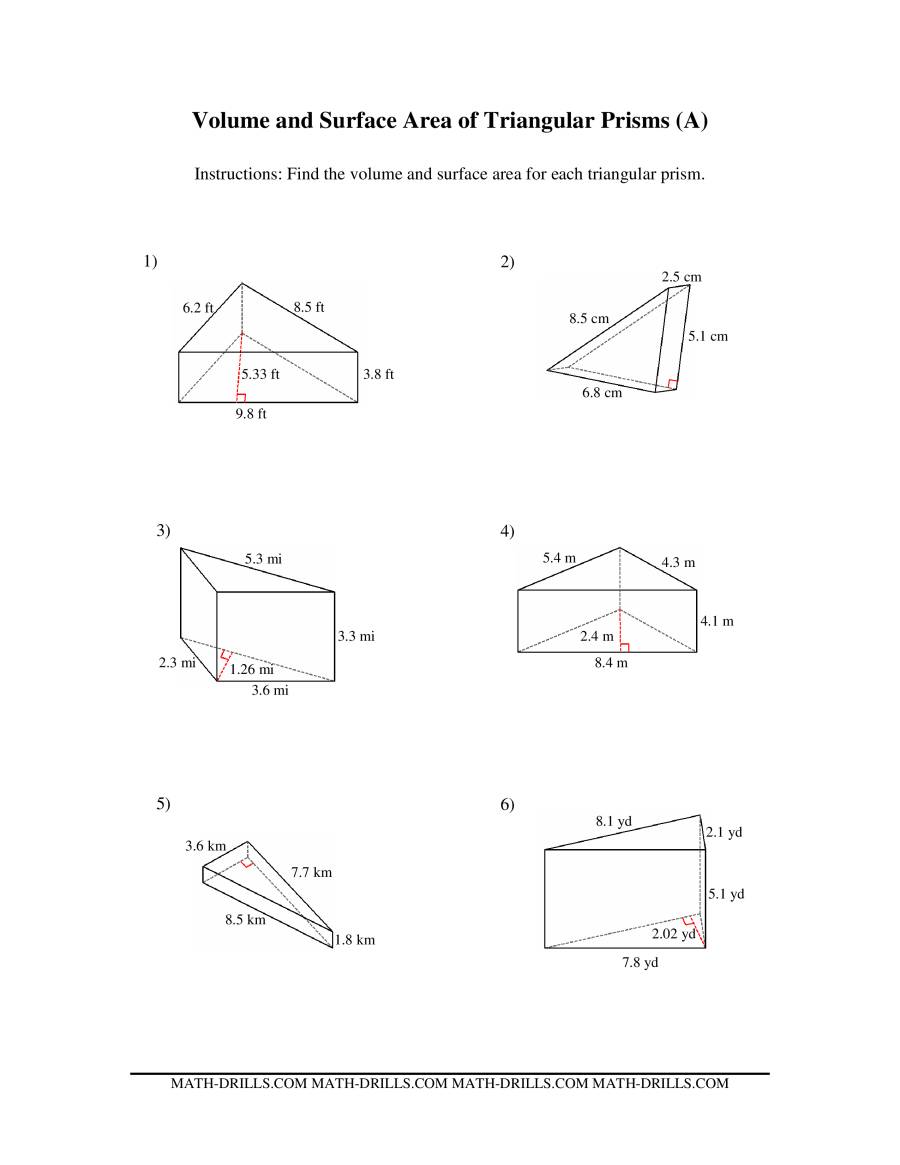
Step 3 :
Find base area.
Area of trapezoid with bases of lengths b₁ and b₂ and height h.
Base area (b) = (1/2) x (b₁ + b₂)h
Base area (b) = (1/2) x (6 + 4)4
Base area = 20 sq.ft
Step 4 :
Find volume of the prism.
V = b x h
V = 20 x 9
V = 180 cubic.ft
So, the number of cubic feet of space in Cherise's tent is 180.
Problem 4 :
Allie has two aquariums connected by a small square prism. Find the volume of the double aquarium.
Solution :
Step 1 :
Find the volume of each of the larger aquariums.
Volume Word Problems
Volume = Base area x Height
Volume = (4 x 3) x 3
Volume = 12 x 3
Volume = 36 cubic ft.
Step 2 :
Find the volume of the connecting prism.
Volume = Base area x Height
Volume = (2 x 1) x 1
Volume = 2 x 1
Volume = 2 cubic ft.
Step 3 :
Add the volumes of the three parts of the aquarium.
V = 36 + 36 + 2
V = 74 cubic ft.
The volume of the aquarium is 74 cubic ft.
Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.
If you have any feedback about our math content, please mail us :
v4formath@gmail.com
We always appreciate your feedback.
You can also visit the following web pages on different stuff in math.
ALGEBRA Negative exponents rules COMPETITIVE EXAMS APTITUDE TESTS ONLINE ACT MATH ONLINE TEST TRANSFORMATIONS OF FUNCTIONS ORDER OF OPERATIONS WORKSHEETS | TRIGONOMETRY Trigonometric identities MENSURATION GEOMETRY ANALYTICAL GEOMETRY CALCULATORS Analytical geometry calculators MATH FOR KIDS LIFE MATHEMATICS SYMMETRY CONVERSIONS |
WORD PROBLEMS
HCF and LCM word problems
Word problems on simple equations
Word problems on linear equations
Trigonometry word problems
Word problems on mixed fractrions
OTHER TOPICS
Ratio and proportion shortcuts
Converting repeating decimals in to fractions
SBI!
In these lessons, we will learn how to
- calculate the surface area of solid cylinders.
- calculate the surface area of hollow cylinders or tubes or pipes.
- solve word problems about cylinders.
- calculate the surface area of cylinders using nets.
Surface Area of a Solid Cylinder
A cylinder is a solid that has two parallel faces which are congruent circles. These faces form the bases of the cylinder. The cylinder has one curved surface. The height of the cylinder is the perpendicular distance between the two bases.The net of a solid cylinder consists of 2 circles and one rectangle. The curved surface opens up to form a rectangle.
Surface area = 2 × area of circle + area of rectangle
Surface Area = 2πr2 + 2πrh = 2πr(r + h)
where r is the radius and h is the height.
Worksheets
Surface Area Word Problems 7th Grade Math
Calculate the volume of cylindersCalculate the surface area of cylinders
Volume and surface area of cylinders
Surface area of cylinders and pipes
Example:
The diameter of the base of a cylinder is 12 cm and the height is 8 cm. Find the surface area of the solid cylinder.
Solution:
Radius = 6 cmSurface area = 2πr (r + h)
=
= 528 cm2How to derive and use the formula of the surface area of a cylinder?
- Show Step-by-step Solutions
Find the surface area of a cylinder with r = 18in, h = 17in. How to calculate the surface area of a cylinder in terms of pi?
- Show Step-by-step Solutions
Surface area of a hollow cylinder
Sometimes you may be required to calculate the total surface area of a hollow cylinder or tube or pipe.
Total surface area of hollow cylinder
= area of internal curved surface + area of external curved surface + area of the two rings
Example:
The figure shows a section of a metal pipe. Given the internal radius of the pipe is 2 cm, the external radius is 2.4 cm and the length of the pipe is 10 cm. Find the total surface area of the pipe
Solution:
r = 2, R = 2.4, h = 10
Total surface area of pipe= area of internal surface + area of external surface + area of the two rings
= 2πrh + 2πRh + 2(πR2– πr2)
= (2π × 2 × 10) + (2π × 2.4 × 10) + (2 × (2.42π – 22π))
= 40π + 48π + 3.52π
= 91.52π
= 91.52 × 3.142
= 287.56 cm2
Word problems about cylinders
Problem:How many square feet of metal are used to make the can?
- Show Step-by-step Solutions
Find the surface area of a cylinder without the lid.
Surface Area of cylinder using nets
Use the net of a cylinder to determine its volume and surface area.Surface Area Word Problems 6th Grade
- Show Step-by-step Solutions
Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.
7th Grade Math Problems And Answers
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.